How to solve simultaneous linear equations within 10s

 Magic Tricks For Simultaneous Linear Equations

Special sutra of Vedic Mathematics – ‘Sunyam Anyat’

 

What Are Simultaneous Linear Equations?

Simultaneous linear equations with two variables.

Let’s say x and y are two variables. Since there are two variables in the equation we cannot solve it by itself. We need another equation with the same variable values to find the answer. When these two equations are solved together we get the values of the variables x and y.

Vedic approach to solve simultaneous linear equations.

In this method we will not be forming new equations but instead we will calculate the values of x and y with the given equations only. The value of the variables x and y will be expressed in the form of numerator upon denominator.

x = Numerator/ Denominator and y = Numerator/ Denominator

If we obtain the value of either x or y then the value of the other variable can easily be obtained by substitution.

Examples:

Find the values of x and y for the equations 2x + 4y = 10 and 3x + 2y = 11.

As mentioned, we will calculate the value of x as numerator upon denominator. The value of numerator will be:

2x + 4y = 10

3x + 2y = 11

The numerator is obtained by cross-multiplying (4 × 11) and subtracting from it the cross product of (2 × 10).

So, x = Numerator/ Denominator

        = (4x 11) – (2×10) / Denominator

        = (44 -20) / Denominator

        = 24/ Denominator

Next, we will calculate the value of the denominator.

2x + 4y = 10

3x + 2y = 11

The denominator is obtained by cross-multiplying (4 x 3) and subtracting from it the cross product of (2 x 2).

x = Numerator/ Denominator

  = 24/ ( 4×3) – (2 x 2)

  = 24/ (12 – 4)

  = 24/ 8

  = 3

Thus, we have obtained the value of x as 3. 

Now, we will substitute the value of x in the equation 2x + 4y = 10, in order to get the value of y.

2(3) + 4y = 10

y = (10-6) / 4

  = 1

Therefore, the values of x and y are 3 and 1 respectively.

Let’s try another example.

Solve the equations 2x + y = 5 and 3x – 4y = 2.

 

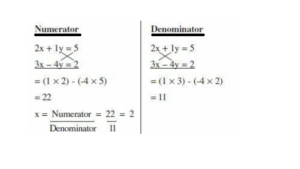
On substituting the value of x = 2 in equation (1) we get the value of y as 1. The solution set is (x=2, y=1).

Let’s try another example, by calculating the value of y.

Solve the equations 6x + 4y = 50 and 5x + 5y = 50.

In this case, we have obtained the value of y as 5. 

We substitute the value of y in equation (1) and get the value of x as 5. 

Thus, the solution is (5, 5).

Special sutra of Vedic Mathematics called the ‘Sunyam Anyat’ which says ‘If one is in ratio, the other is zero.’

Let’s understand by an example,

5x + 8y = 40

10x + 11y = 80

In the above case, the x co-efficient s are in the ratio of 5/10 = 1:2 and the constants terms are also in the ratio of 40/ 80 = 1:2.

Now, our sutra says that ‘if one (variable) is in ratio, the other one (the other variable) is zero.’

In this case, we see that the variable x is in ratio with the constant terms and therefore the other variable y, is zero.

Thus, value of y is zero.

Put the value of y in the above equation to get the value of x.

If we take equation (1) and substitute the value of y as zero, we have 5x = 40 and hence x= 8.

Keep reading…keep learning…