
Two 5-Seconds tricks for splitting the middle term
There is nothing more basic than a quadratic equation composed of degree two variables, constants, and integers. A variable in mathematics is a symbol, or letter, that lacks a value. The term “constant” also applies when its value is fixed. Here we will learn how to use two 5-seconds tricks for splitting the middle term. Use the division method to get two factors, and if the coefficient is larger, use the prime factorization approach, which is the second method. Let’s learn with examples.
Factorize 6x2 – 7x -20
To find the two factors, I will first apply the division method.
Here, product of 6 and 20= 6 x 20 = 120
I need to locate two factors out of 120 so that middle term 7 can be obtained by adding or subtracting them.
In order to obtain two factors, I will create a table with two rows. In the first row, I will attempt all the factors from 1 to 9 that divide 120 entirely. In the second row, I will write their quotient. After that, I’ll see which combination of the two criteria offers me a medium term. Watch this.
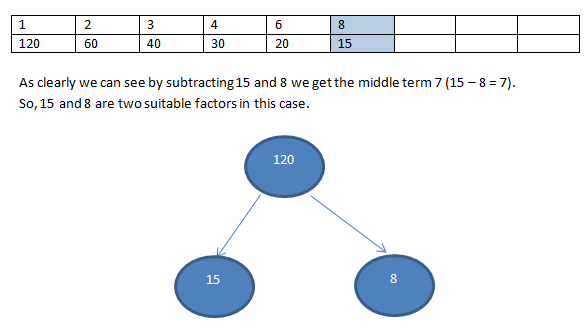
6x2 – 7x -20
= 6x2 – (15x-8x) -20
= 6x2 – 15x + 8x -20
= 3x(2x – 5) +4 (2x – 5)
= (2x -5) (3x+4)
Splitting mid term using prime factorization method
Factorize 6x2 – 7x -20
Let’s get two factors using prime factorization method.
Product of first and last term = 6 x 20
Prime factors of 6 = 2 x 3
Prime factors of 20 = 2 x 2 x 5
I will divide these factors into two groups and try to get the middle term by adding or subtracting.
6 x 20 = [ 2 x3 ] x [ 2 x 2 x 5] ——————- (A)
= [2 x 3] x [2 x 10] (I started by making a pair in each box, then I left the smaller number in the second box and multiplied the remaining numbers to produce two multiples.)
= [6] [20] (this will not give me middle term 7)
Now, I’m going to rewrite the factors by grouping the larger factors together:
[3 x 10] [2 x 2] = [30] [ 4] ( This is also not working, cannot get 7 middle term by adding or subtracting 30 & 4).
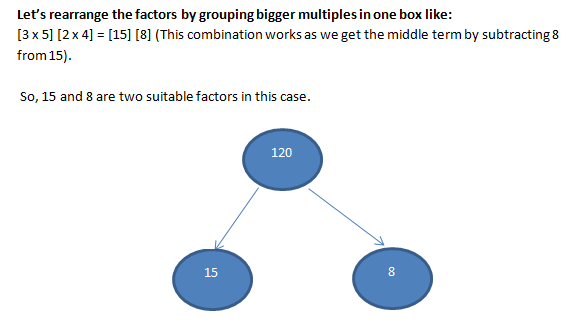
6x2 – 7x -20
= 6x2 – (15x-8x) -20
= 6x2 – 15x + 8x -20
= 3x(2x – 5) +4 (2x – 5)
= (2x -5) (3x+4)
Therefore, I advise using the prime factorization approach to obtain two factors if your coefficient is larger. Let’s look at an additional case with a larger coefficient.
Q3: 11x2 – 54x + 63
[11] x [63] = [11] [ 3 x 3 x 7] = [11] [ 3 x 21] = [11 x 3] [21] = [33] [21] ( This will give the middle term by adding them).
11x2 – 54x + 63
= 11x2 – (33x+21x) + 63
= 11x2 – 33x -21x + 63
= 11x ( x -3) -21 (x -3)
= (x-3) (11x -21)
